Distance-Drawdown Graphical
Distance Drawdown (Graphical) Method
View | Distance-Drawdown Graph
See Also:
Distance Drawdown (Automatic) Method
This method is used for tests where drawdown is measured at the same time intervals in two or more observation wells different distances from the well. The screen will display a graph of the drawdown in each well arithmetically on the vertical axis, and the logarithm of the distance from the pumping well to the observation well on the horizontal axis.
If the assumptions for the Theis solution are met, the points will plot a straight line. A best fit line is drawn through the points. The slope of the line is used to estimate the aquifer transmissivity and storativity. The intersection of the line with the horizontal axis (drawdown = 0) is an estimate of the radius of influence.
Calculation results are displayed in moveable text boxes.
Selecting the Trial
The distance-drawdown graph can be displayed for only one time measurement. By default, the final time measurement is displayed first. Two buttons appear at the bottom of the screen. The Previous Trial button changes the graph display to the next earlier time measurement. The Next Trial button changes the display to the next later time measurement. The down arrow on the keyboard acts the same as the Previous Trial button. The up arrow on the keyboard acts the same as the Next Trial button.
Transmissivity
Transmissivity is determined from the equation:
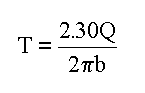
where:
Q is pumping rate
b is the slope of the graph of drawdown vs. log of radius (or drawdown per log cycle radius)
Radius of Influence
Radius of Influence is determined by the equation:
![]()
where:
a is the drawdown at log of radius = 0
b is the slope of the distance drawdown graph
Storativity
Storativity is determined by the equation:
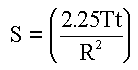
where:
t is the time since pumping started
R is the radius of influence of the test as determined above
T is the estimated transmissivity of the aquifer.